...distance
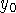Important point: dy is entirely independent of y! Think of it as a step of size tex2html_wrap_inline690 , which can be chosen arbitrarily, with no relation to the place tex2html_wrap_inline684 from which you step. 
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
...functional
L can contain t explicitly if the potential V is time-dependent. We ignore this in the following. 
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
...equation.
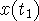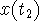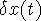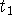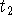Since Hamilton instructs us to solve a boundary value problem, with tex2html_wrap_inline1012 and tex2html_wrap_inline1014 fixed, the variation tex2html_wrap_inline1016 is fixed to be zero at tex2html_wrap_inline986 and tex2html_wrap_inline990 . Thus x in (33) is not a boundary point, and there are no boundary terms to worry about. 
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
...solution.
Just change the boundary conditions on 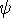tex2html_wrap_inline1064 , for example. A more interesting alternative is to add tex2html_wrap_inline1094 to the energy (50). 
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.